This website uses cookies to improve your experience. We'll assume you're ok with this, but you can opt-out if you wish.

Talking math with your kids

Five fun things to do with 21st Century Pattern Blocks

by

I wrote last week about the release of 21st Century Pattern Blocks on a much larger scale than before. This week, I want to share five fun math adventures that begin with these blocks.

1.Scaling

Which 21st Century Pattern Blocks can you use (any number of) themselves to make a larger version of themselves?

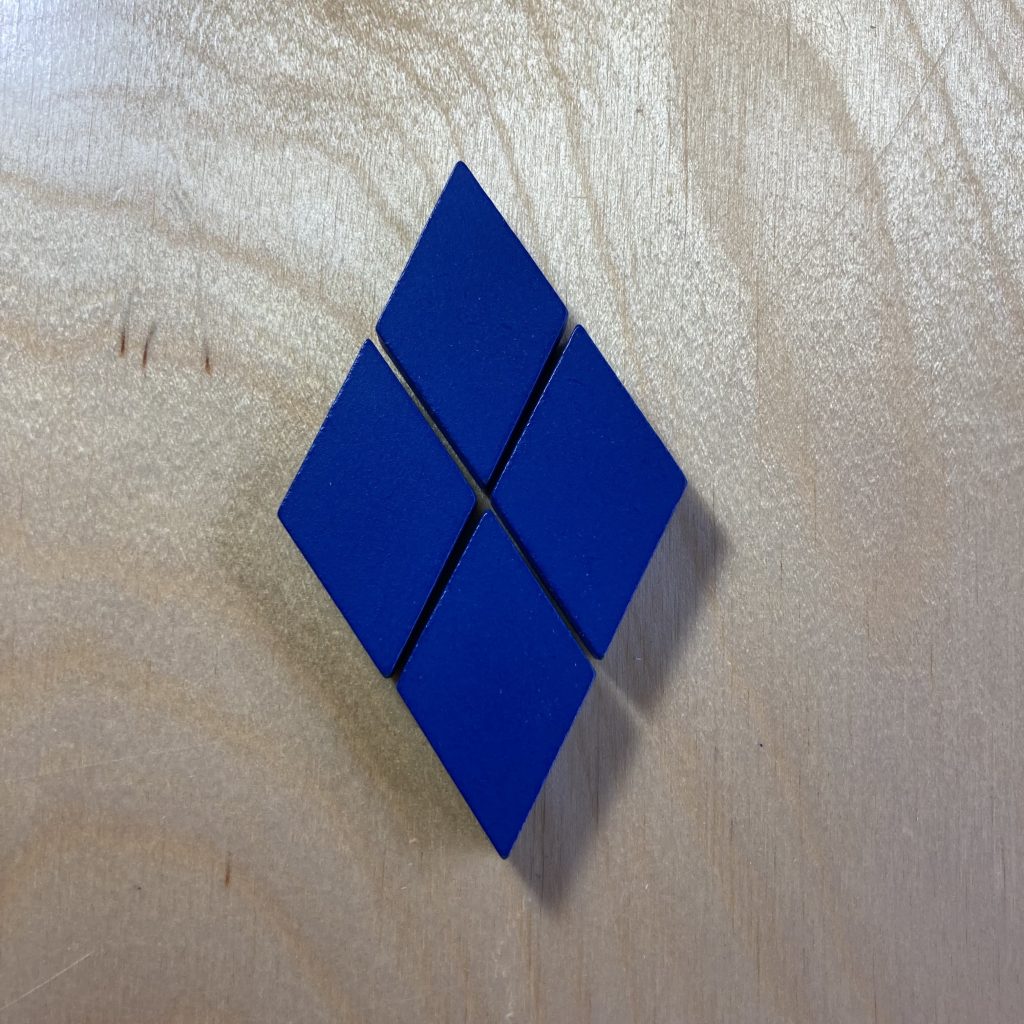
Four small blue diamonds tiled to make a larger, similar diamond.

Which blocks can you make larger versions of, using OTHER blocks? 

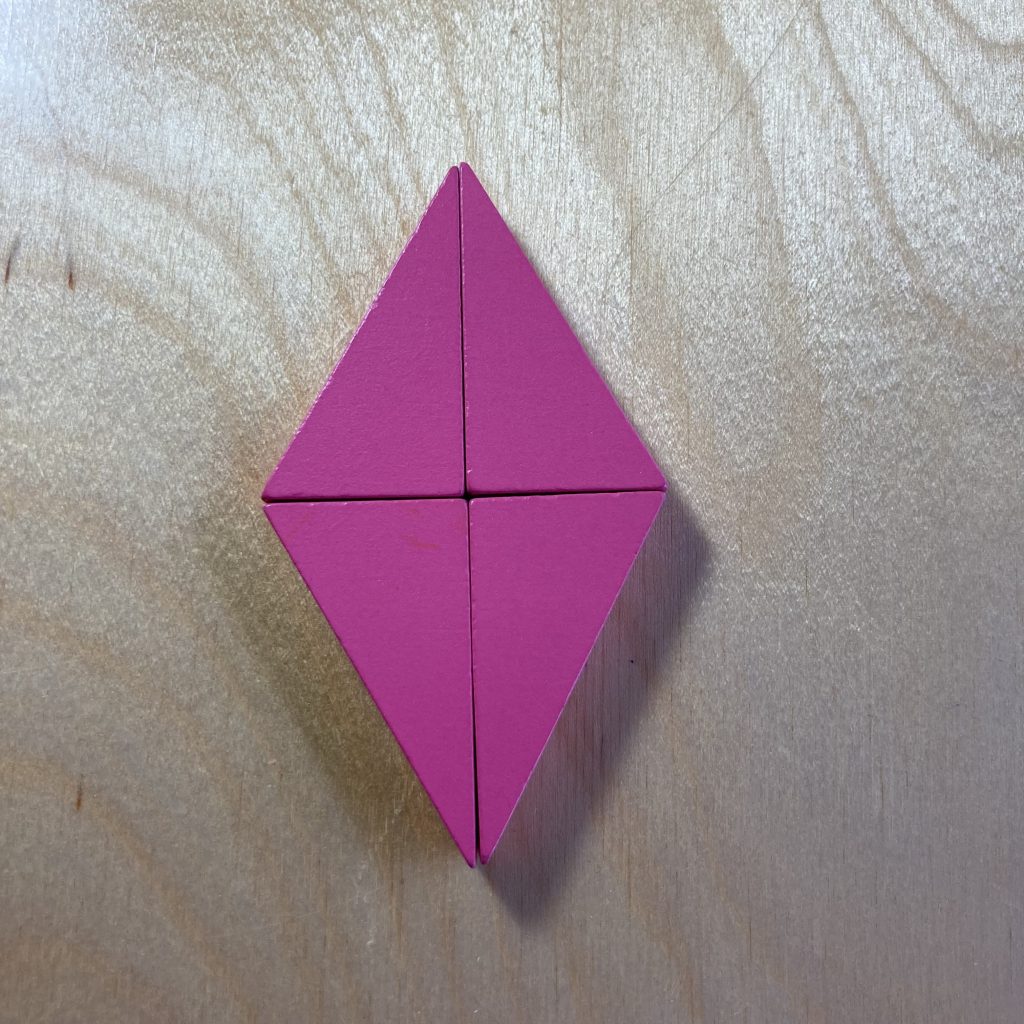
Four pink right triangles tiled to make a diamond (rhombus)

Are there any blocks you can’t make a scaled copy of, using triangles?

2. More Scaling

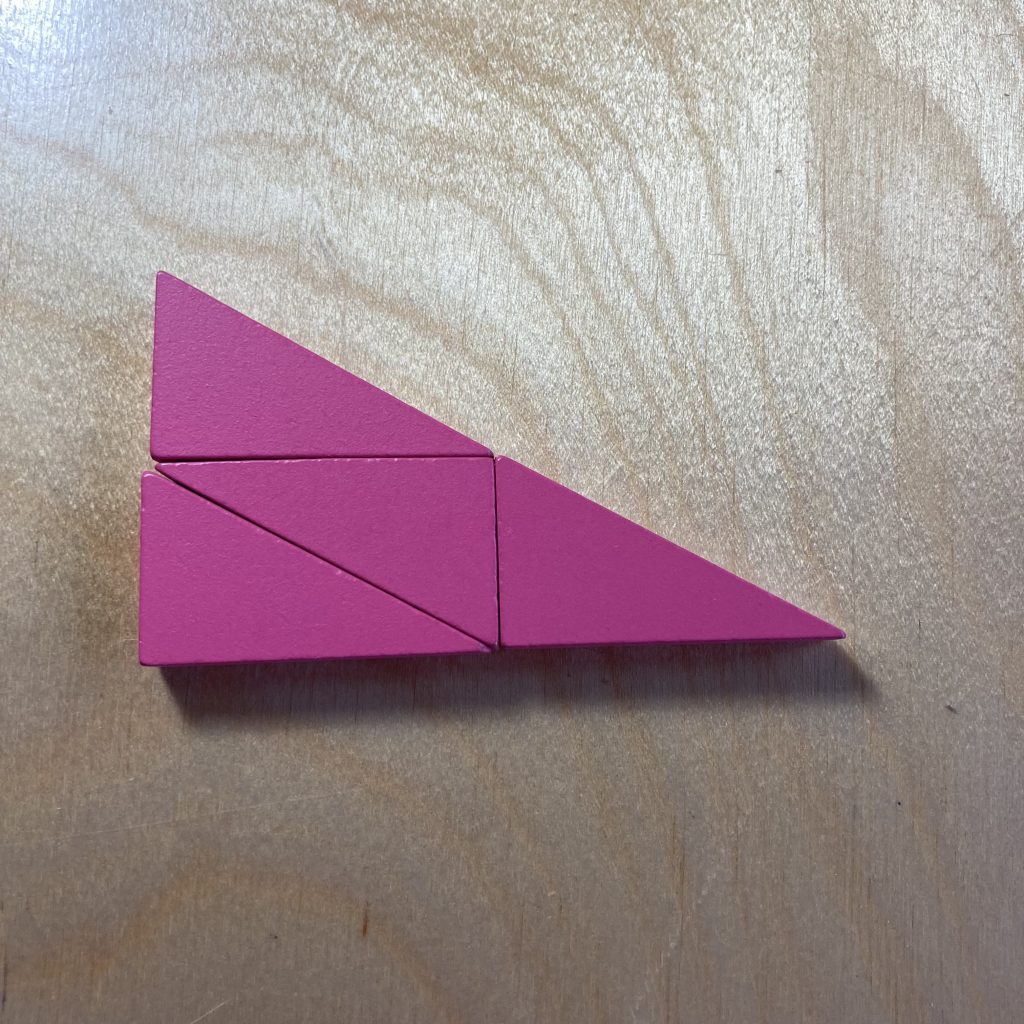
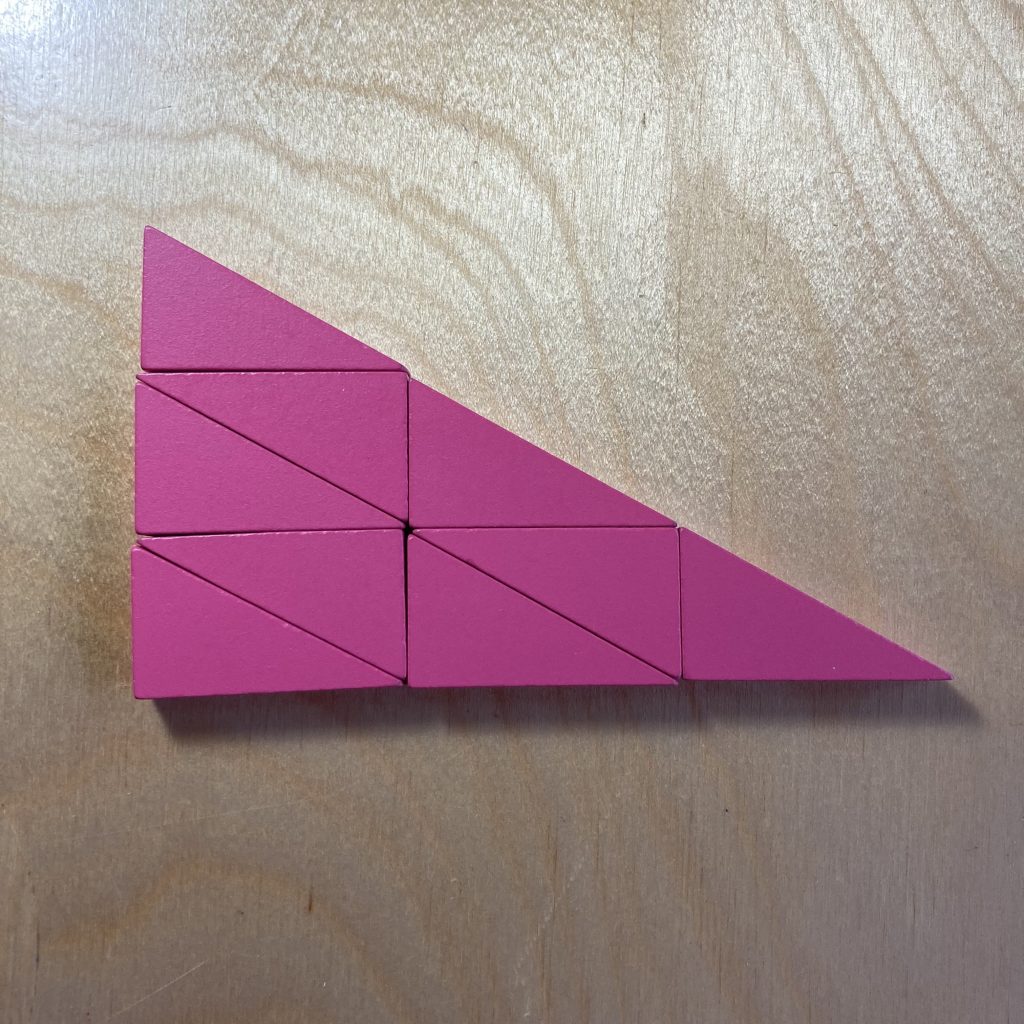
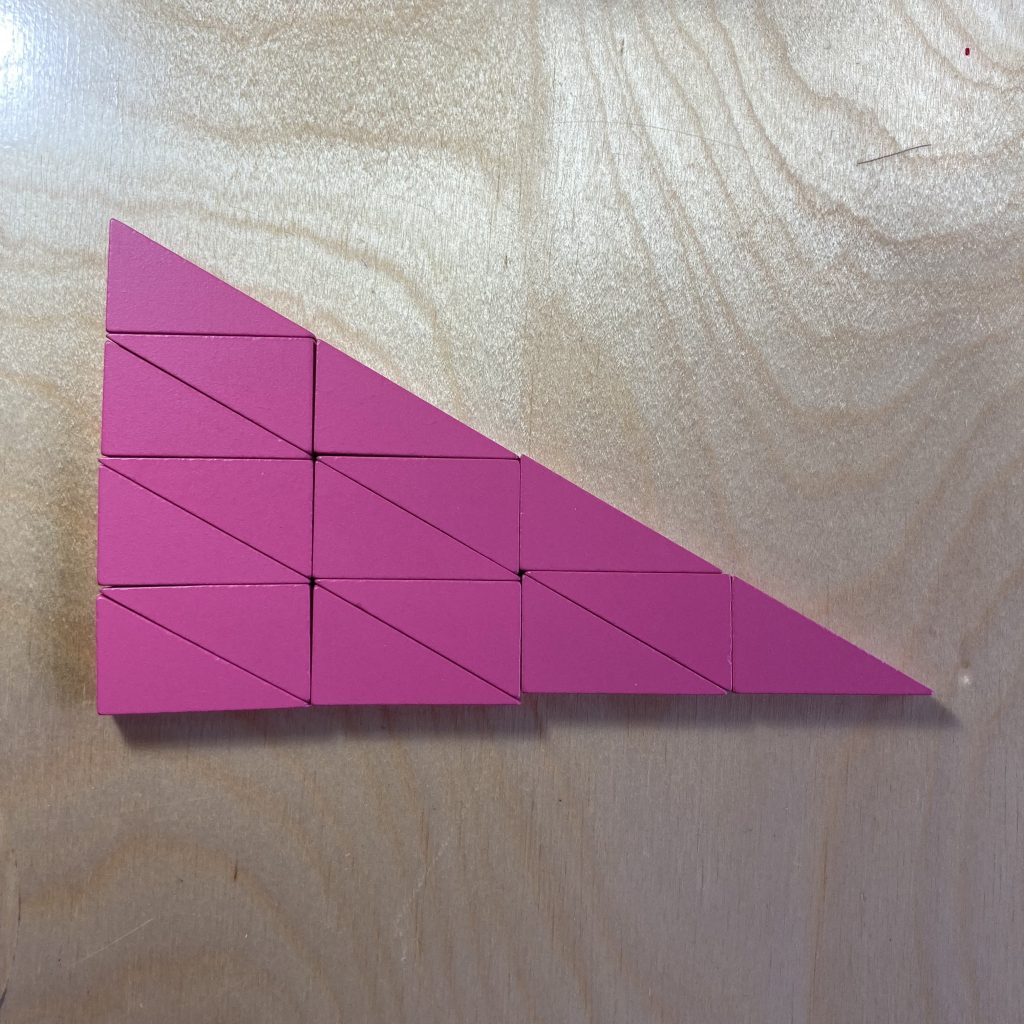
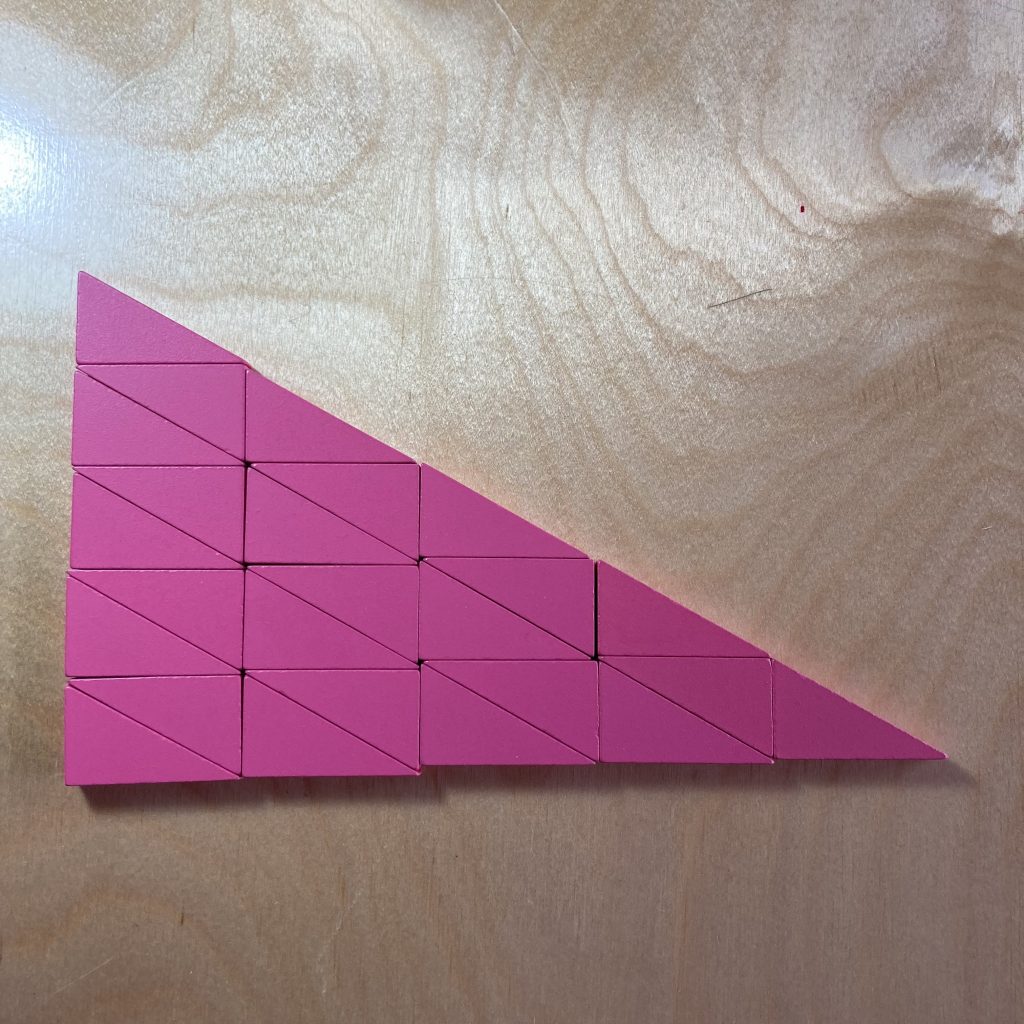
All triangles have the property that you can make a larger, congruent version of themselves using four of the original size (and also 9, 16, and all the squares). 

  • Four pink right triangles tiled to make a larger, similar triangle.
  • Nine pink right triangles tiled to make a larger, similar triangle.
  • Sixteen pink right triangles tiled to make a larger, similar triangle.
  • Twenty-five pink right triangles tiled to make a larger, similar triangle.

NOT ALL triangles have the property that you can do this with three. This triangle does. 

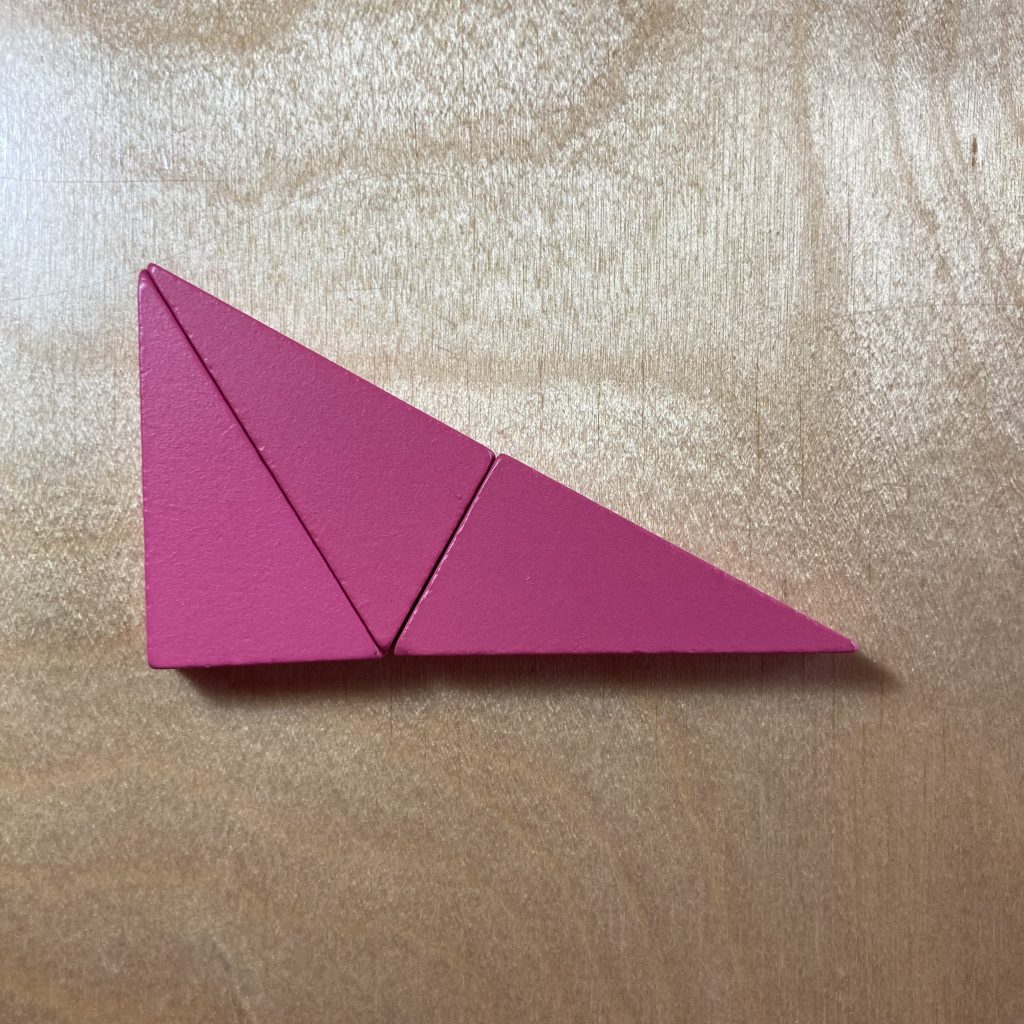
Three pink right triangles tiled to make a larger, similar triangle.

Try it. 

What other numbers of triangles can you use to scale this triangle up, besides 3 and the squares? Can you design a new triangle that you can scale up by a factor other than 3 or the squares? What would a triangle look like that can be scaled up with TWO copies of itself? Or FIVE? Are there any numbers for which there is no scaling triangle?

3. Symmetry

The triangle is asymmetric. 

You can spin the triangle in the center of this image to match any of the ones that surround it. Let’s call this a clockwise-triangle because the triangles are all pointing around a clockwise circle.

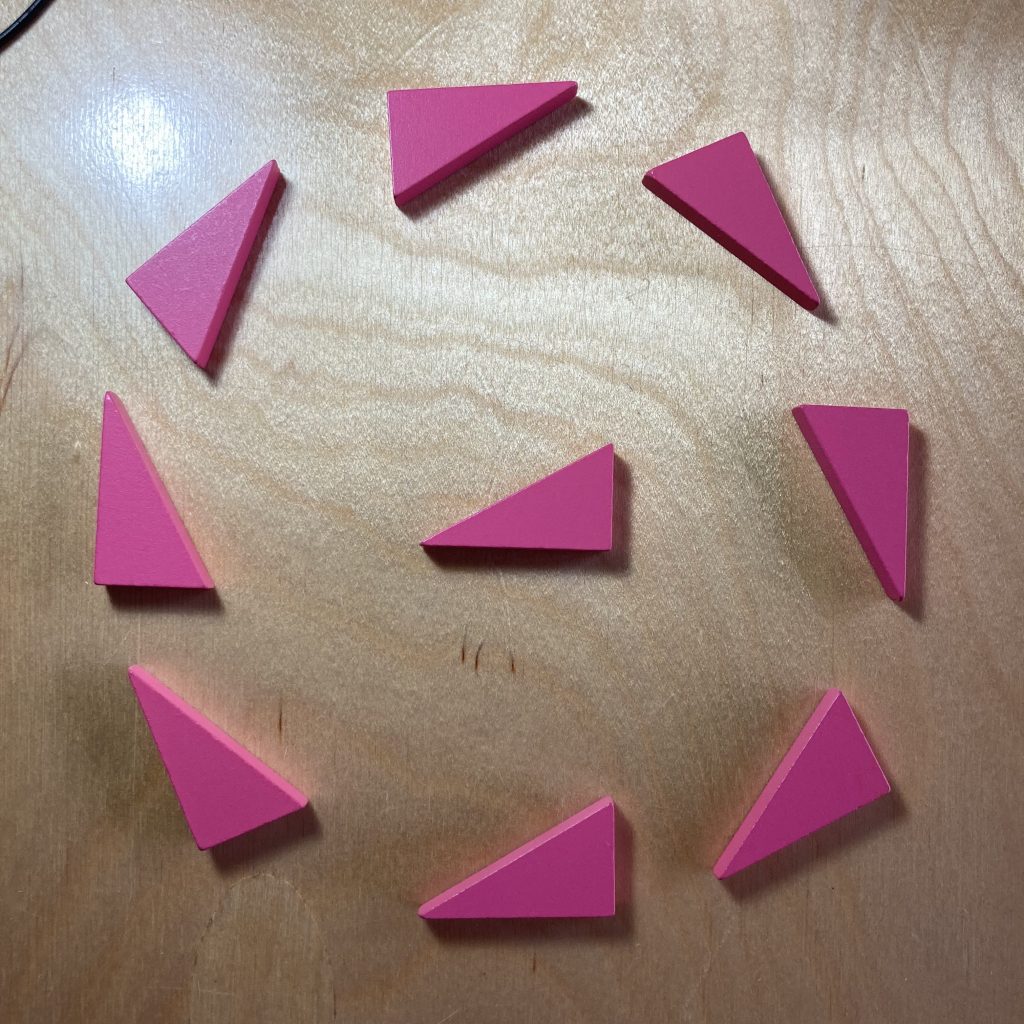
A single pink right triangle is surrounded by eight regularly spaced identical triangles in a circle, each pointing clockwise around the circle.

In this second image, you’ll need to flip the triangle in order to match the counter-clockwise facing circles surrounding it.

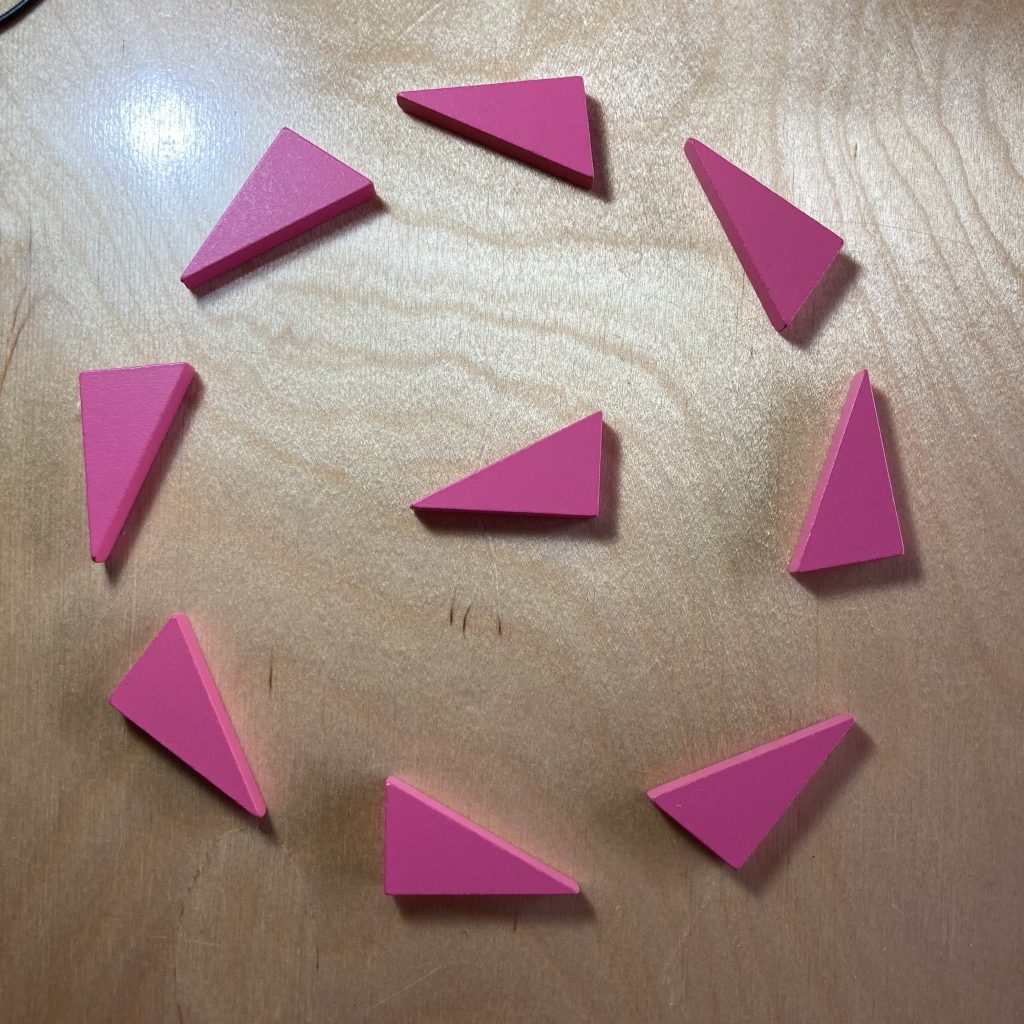
A single pink right triangle is surrounded by eight regularly spaced identical triangles in a circle, each pointing counter-clockwise around the circle.

Here’s a fun little game to play with that asymmetry. 

Select one triangle and open mirrors to the angle where you see three triangles—the real one and two reflections.

one pink right triangle, lying on a polished wooden table, pointing to the left. There are two hinged mirrors, opened to an obtuse angle, and reflecting the triangle in each

Notice that the other two are opposite handed-ness as your original. In this case, the original is a clockwise triangle. The other two are counterclockwise. 

Now close a bit until you see four triangles, and verify that you’ve got two of each. The new one that appears is pointing the same direction around the clock as the original. 

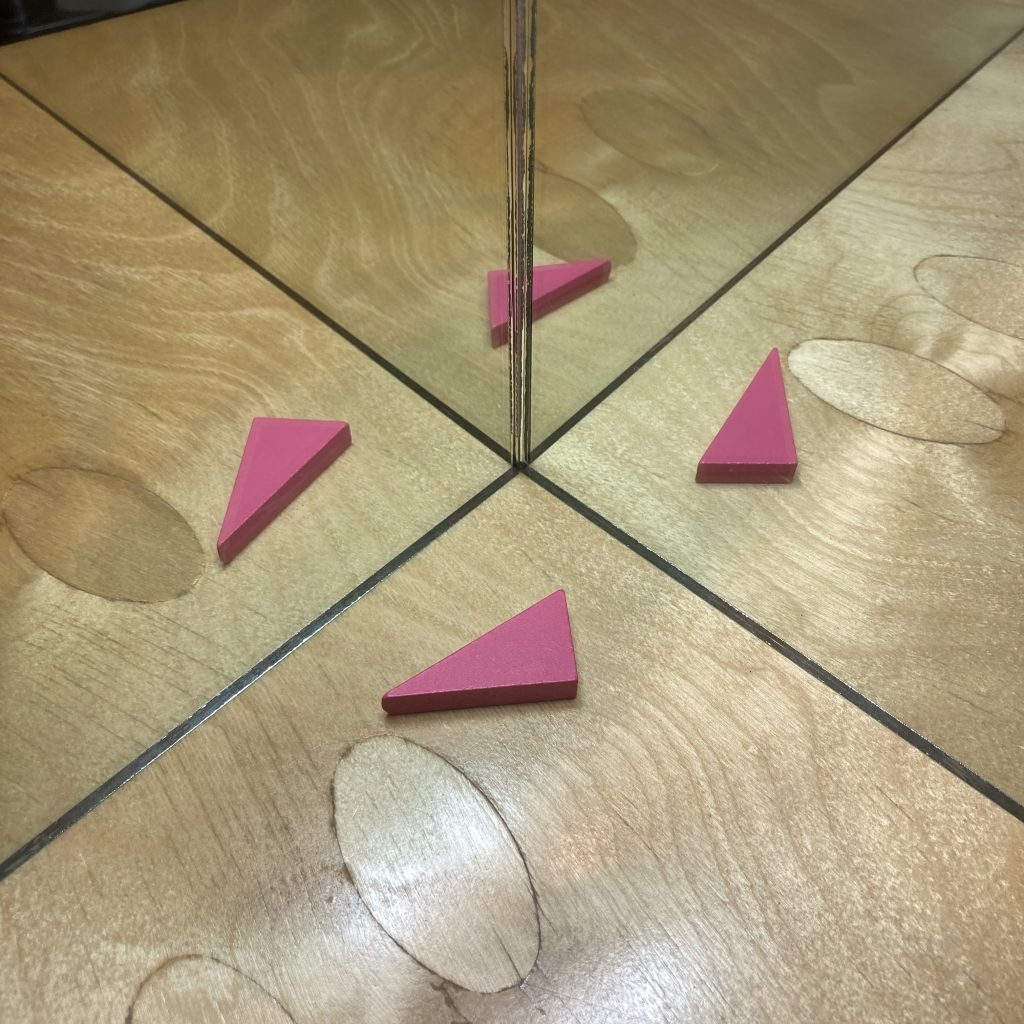
one pink right triangle, lying on a polished wooden table, pointing to the left. There are two hinged mirrors, opened to a right angle, and reflecting the triangle in each. There is a fourth triangle in the reflections of the reflections

Before going to five, make a prediction. Which direction will it be facing? Continue and conjecture how things will play out as you close the mirrors and make more triangles. If you had 11 triangles (the real one, plus 10 in the mirrors), would you have an extra clockwise or an extra counter-clockwise triangle?

4. Area and Perimeter

Put the blocks in order according to area, from smallest to largest. Then do the same thing by perimeter. What differences do you notice between those orderings? Which blocks were most difficult to measure or order by area? by perimeter?

(No images for this one, because they’d give away the fun!)

5. Compare Side Lengths

If the shortest side of the triangle is one unit, then the longest side is two units. You can verify that by lining the triangles up next to each other. You’ll cover the same length with 2 short sides and one long side. And also 4 short sides and 2 long sides cover the same length. 

Two pink right triangles on top, their hypotenuses lined up against the short sides of four identical pink triangles beneath, demonstrating that two of the hypotenuse lengths equal four of the short side lengths.

What about the medium side length? How many short (or long) sides can you use to cover the same length as some number of the medium sides?

Follow Talking Math With Your Kids on WordPress.com