After seeing Hana Murray’s lovely study of rectangles made from 21st Century Pattern Blocks, I started playing and noticed that many of them seemed to involve the small green triangles.
Pretty soon, I had formed a conjecture.
Using copies of these at least two different types of these five blocks (concave hexagon, grey dart, pink triangle, teal kite, blue rhombus), it is impossible to make a rectangle.

A little while later, I had a counterexample.
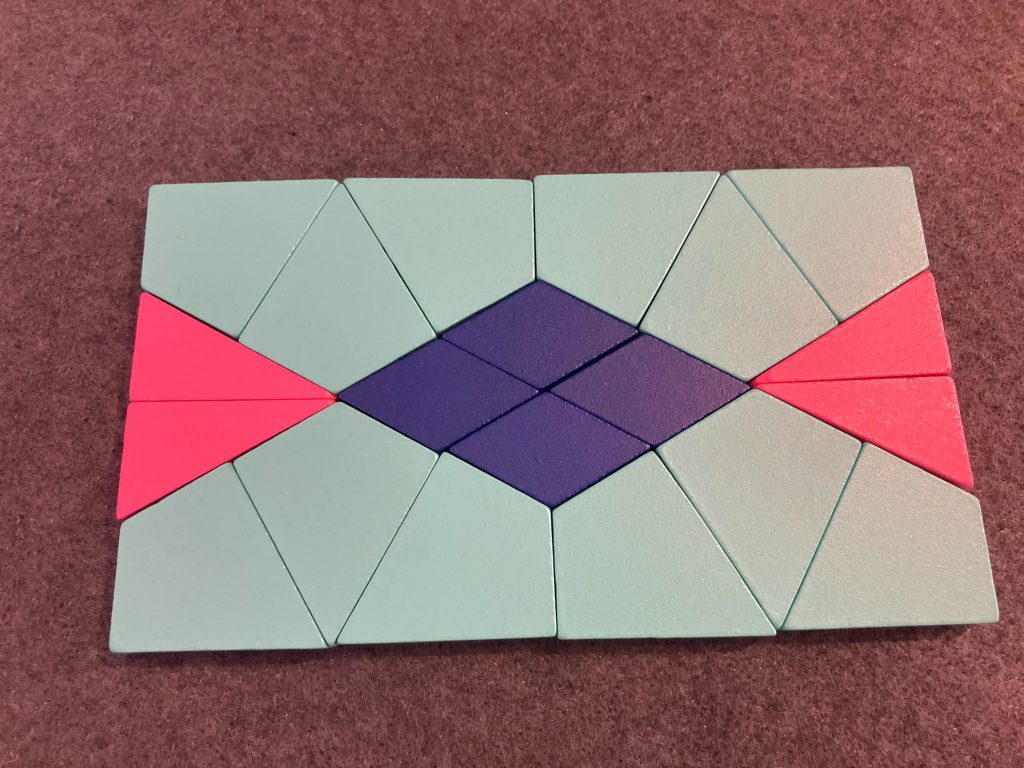
Now I have a new conjecture.
Using copies of these at least two different types of these five blocks (concave hexagon, grey dart, pink triangle, teal kite, blue rhombus), the rectangle above is the smallest possible rectangle.
If you or the children in your life are inclined to work on that conjecture, I welcome both your arguments and your counterexamples over on Twitter (#21stCenturyPB) or by email: christopher@talkingmathwithkids.com
