I wrote about two competing theories recently. Each is an answer the question Why do you never see a square on the rectangle page of a shapes book for young children?
That question stands in for lots of other specific questions, such as Why do counting books tend to stop at ten? and Why are all shapes-book triangles equilateral and oriented on a base?

Yet this is its only triangle.
Together, these questions stand in for a more general one, Why are math resources for young children so much more simplistic than the stories written for them?
All of these questions gloss over nuance. Surely there is a square on a rectangle page somewhere! Excellent counting resources do exist, such Anno’s Counting Book. But these examples are rare. Mathematical resources for young children are generally limited in some very specific ways and these limitations are prevalent both in and out of school.
The two competing theories are these:
- People involved in producing these resources don’t know the math.
- People involved in producing these resources don’t think it matters whether young children see excellent math.
Of course each can be true to some extent; it’s not all one or the other. I have been attending to how squares and rectangles get treated in materials and instruction for young children for many years, and I have collected evidence that the second theory is quite often true.
Just one story among many took place at a large conference. There I made a new acquaintance; a person in a position of great influence at a corporation that puts a lot of math objects into American elementary classrooms.
Being in talk-prep mode, I handed this person a Post-It and asked them to draw me a hexagon. They drew one of those in the image below, I forget which one (but not the trapezoid! That’s a whole different story!)
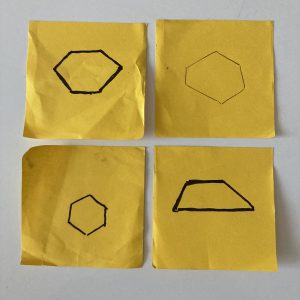
“Isn’t it interesting,” I suggested, “that your hexagon looks a lot like these other hexagons people drew earlier today, and not like any of the other ways a six-sided polygon can look?”
My new acquaintance’s reply was that “hexagon” has a different meaning in everyday English than it does in formal mathematics. In everyday English, hexagon is taken to mean regular hexagon; something that looks like a particular iconic shape. Words have different meanings in different contexts, and so it is with hexagon.
“But it doesn’t have to be that way!” I replied. “What if the resources offered to children, families, and early elementary teachers showed a variety of hexagons?” At which point, I pulled out a hexagon puzzle I just happened to have handy.

My conversation partner was unmoved.
“OK,” I said. “Right. Hexagon isn’t a big or important concept in mathematics. I get that. I think it matters as an example; I get that you don’t.
“So let me tell you about my seventh-grade classroom from back in the day. When I taught middle school, I had them look for all of the rectangles with a fixed perimeter and compare their areas. The one with the biggest area is the square, but my students would always skip over that one because they had been told—either explicitly or by example—so many times in their 12-to-13 years of life that rectangles have two long sides and two short sides. And also that hexagons are regular, and that triangles are short, squat, and point upwards.”
“I might just be glad,” my conversation partner replied, “that they knew what rectangles are.”
Dearest Reader, I might have gotten a little worked up at this point.
“You’re telling me,” I replied, “that as [influential position] at [influential education corporation], that’s your bar for seventh graders and geometry?”
They walked their words back a bit. They told me they had a mathematics degree. We parted ways with an understanding that I was pretty worked up about something that doesn’t matter very much.
In another—much lower stakes—conversation with a friend at a bookstore, I pointed out the lack of a relationship between squares and rectangles in this book:



“Yeah, I see it,” my friend replied. “Maybe it’s just too hard to do that right? Too many words or something.”
I have given it some thought. Here’s one solution. Swap the order and add ten words.


Anyway, the point is that that we can do better.
We can give children and caregivers better math for better learning. We just have to believe that we can.
We have to want to.
