Pattern Machines: What They Are and Why You Need One

If you have children in your life who are between the ages of four and ten, you need a Pattern Machine.

I’m going to tell you what a Pattern Machine is, and then tell you why you need one (or more).

What Is a Pattern Machine?

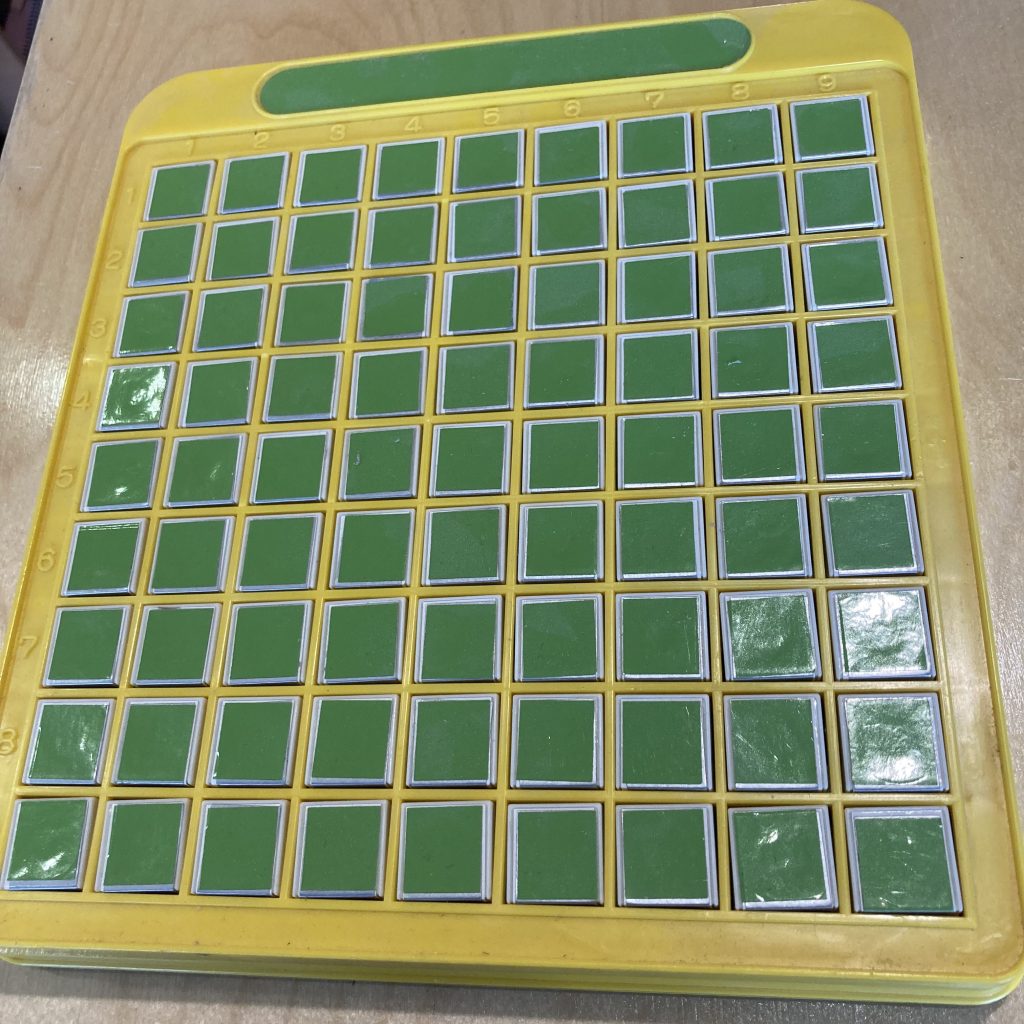
A Pattern Machine starts out as a Multiplication Machine, which are sold by Lakeshore Learning. Essentially a set of mechanical flash cards, a Multiplication Machine is a set of 9 rows of 9 clicky buttons function like the end of a ball point pen. Click once to pop it up; click again to pop it down.

On top of each button is a single-digit multiplication, such as 1×1 in the upper left corner, 6×4 somewhere in the middle, and 9×9 down in the lower right. On the front of each button—visible only when you pop up the button—is the corresponding product (1, 24, and 81 in the cases of the buttons I’ve just mentioned). 

There also exists an Addition Machine, which works exactly the same way, but with sums replacing the products. Also, there are Subtraction and Division Machines, which are very badly designed. I’ll write about them another time.

The problem with Multiplication Machines is that the least fun way to use them is the way that they were intended. That intention is parallel to flash cards—look at a button (analogous to the front of a flash card), think of what the product is, then punch the button to check yourself (this is like flipping over the flash card). 

Here is the advertising copy from the Lakeshore Learning website:

Our self-checking Multiplication Machine makes math so simple, kids can teach themselves! Children just press the equation buttons…and the answers pop up for immediate reinforcement!

An eight-year-old girl told me on the first-ever day of Math On-A-Stick that she had a Multiplication Machine at home, but she said, “I never use it for math; I draw pictures on it.”

Drawing pictures, making patterns, inventing games, posing problems—these are what children naturally want to do with Multiplication Machines. Each of these activities is more fun than running through a set of flash cards, and is also more mathematically productive than memorizing your multiplication facts.

In order to support productive mathematical activity, you can turn a Multiplication Machine into a Pattern Machine by covering up the multiplication fact on each button with a piece of colorful tape. 3M makes a washi tape that is exactly the right width. Armed with a roll of washi tape and a crafting knife, this transformation takes about 40 minutes—an hour if you go deluxe by using different colors for the front and top of the buttons.

You can reduce that time with practice, and you can cut it in half if you have access to sign vinyl and a vinyl cutter, such as a Cricut. Here are Adobe Illustrator files (file 1 and file 2) you are welcome to use and modify. I have no advice about using a Cricut because I have done all of my own Pattern Machine work on an obsolete vinyl cutter at a maker space that closed last fall. I’m sure I’ll get up to speed with a new machine sometime soon, so check in next fall if you need help or have tips to share. As a bonus, the sign vinyl version is more durable than the washi tape version.

Be sure to cover up the “Multiplication Machine” label while you’re covering the buttons. If you do not, then children (and especially the adults caring for them) will take it as a cue that they’re supposed to figure out how to do multiplication on the machine.

Now that you know what a Pattern Machine is, I’ll tell you why you need one (or more). I want to tell you a thousand reasons, but I’ll limit myself to two reasons, supported by five examples. 

Why Do I Need a Pattern Machine?

Here the two reasons you need a Pattern Machine:

1. They are very fun.
2. Playing with Pattern Machines leads children to do amazing math.

Here are five examples:

1. Rows and columns

Young children don’t know about rows and columns. You can see it in the ways they play with Pattern Machines. A typical four-year-old will want to get all the buttons up, and then put them all down. At first, they will punch buttons at random, as though picking up a bunch of spilled pennies one at a time. But children who persist for five or ten minutes soon start to notice and use the row and column structure of a pattern machine—they’ll punch all the buttons across the top row, then across the next, or maybe up and down the columns. As they do, they’re starting to see a structure that will be useful in learning multiplication, fractions, area, and much more. 

2. Patterns and counting

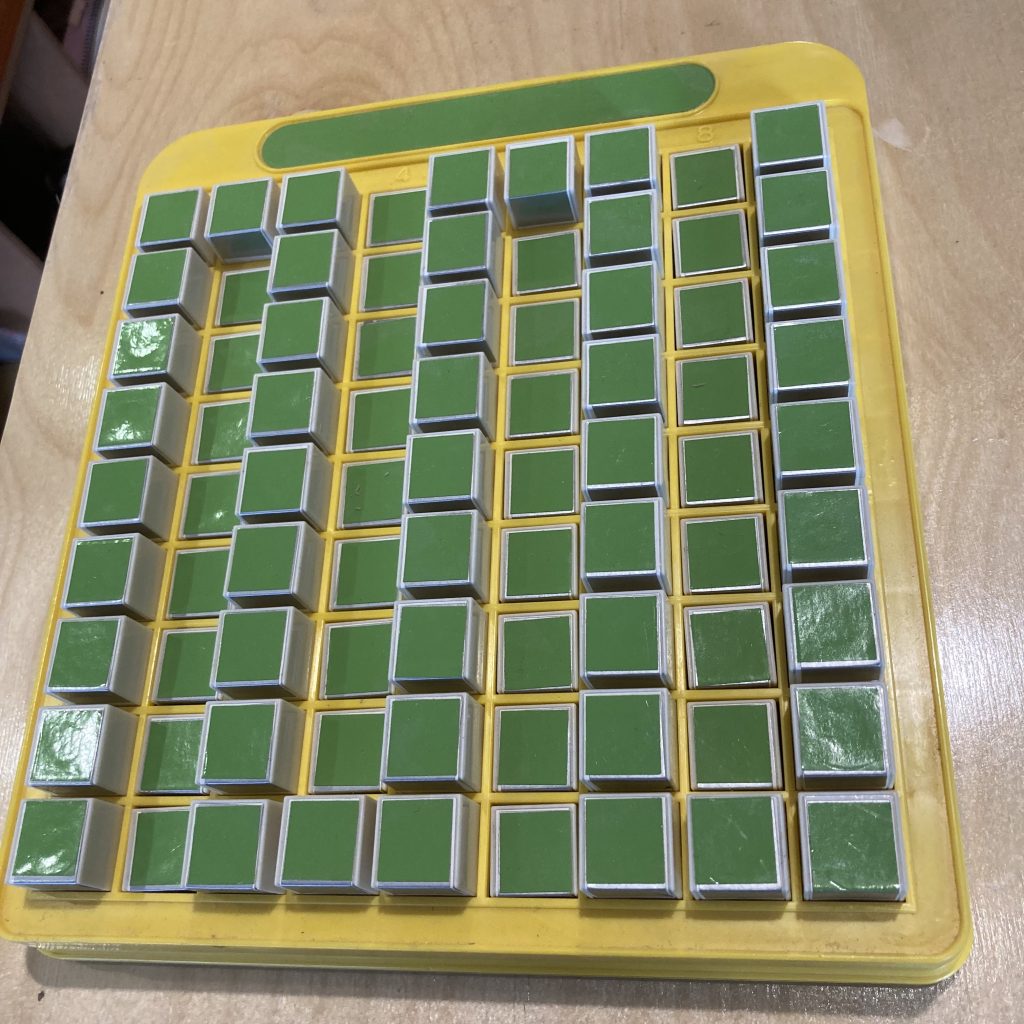
Children make patterns on Pattern Machines without being told; it’s a natural, human thing to want to do. A common pattern is alternating up and down buttons in what children often describe as “a checkerboard”. Making and describing patterns is math, and a couple of simple questions can deepen that math quickly. For example, on the Pattern Machine in the photograph, are there more up buttons or down buttons?

The first instinct is usually that there are the same number—one up for every down. Ask, “How many of each, then?” and most folks will think about 9 rows of 9 buttons, which is 81, which—being odd—means that there can’t be the same number of each. Some people will count each kind next (41 ups and 40 downs), which leads to interesting and challenging counting strategies. 

Others will pursue some kind of argument about whether there are more ups or downs; arguments that don’t require counting the buttons individually. For example, the first row has five ups and four downs—an extra up. The second row is the opposite—four ups and five downs—so it has an extra down. There are five rows with an extra up and only four with an extra down, so there must be one more up than down.

3. Paths

Children sometimes imagine themselves immersed in the world of the Pattern Machine; their fingers becoming little people walking around among the buttons. That can lead to making paths for their imaginary selves to follow, and a natural math question arises: What is the longest path you can make on a Pattern Machine? 

If you take that question seriously, you end up needing to define path more carefully (is a loop a path? can a path branch in more than one direction? etc.) Then a combination of experimentation and analysis can follow, which is an important way for math research to develop. 

Given the following assumptions about paths, the longest known path on a 9×9 Pattern Machine is 53.

• Loops are not allowed
• Paths don’t branch
• Squares on a path connect only along their edges—not at the corners

This is a long path of 49 squares, but it is not the longest possible.

4. Combinations

A five-year-old at Math On-A-Stick one year had a table full of 20 Pattern Machines to herself on a slow weekday. Having just visited the stunt dog show a short distance away, she was interested in imagining pens for the dogs to hang out in between shows. Up buttons formed the walls of each pen, with down buttons representing the space for the dogs. 

Over the course of 45 minutes or so, she made one pen on each machine, and moved to the next machine to make a new, different pen. She had in effect posed a combinatorics problem—How many ways are there to make dog pens on a Pattern Machine? She then started solving this problem by producing new pens, one after another. She began with simple rectangular pens, and proceeded to more complicated shapes. 

In the patterns and counting example, children count buttons. In the dog pen example, the child was counting possibilities. Math is interested in both kinds of counting.

5. Iteration

Repeatedly applying the same idea to new inputs is a common mathematical technique. Place value is an example of iteration, where the idea is groups of 10. Ten ones make one ten. Ten tens make one hundred. Group ten hundreds together to get a new unit—thousands. Fractals are mathematical objects that result of iteration as well.

Another way to describe the place value idea is making units. Ten, hundred, thousand, these are new larger units made by grouping smaller units. Pattern Machines have units too. The first unit is a button, and when children begin to use the rows and columns to make patterns, a new unit may emerge—the row. Remember up above when we were counting up and down buttons? Children sometimes talk about that first row with an extra up as an up row, and to the second row—which has an extra down button—as a down row. Also, they will sometimes notice that these are a sort of opposite of each other. All of this indicates that they have begun to think of a row as its own thing—a sort of unit.

Put nine rows together and you have a new unit—a Pattern Machine. Just as with the rows, children sometimes make two Pattern Machines the opposite of each other. It is very satisfying to nest two opposite Pattern Machines face-to-face with each up button on one machine filling the space left by a down button on the other.

Finally, on one glorious day, we continued this unitizing process by building a Mega Pattern Machine consisting of nine rows of nine Pattern Machines. It was a true mathematical achievement!

Here is an object that seems kind of like a Pattern Machine, but which is not at all a Pattern Machine.

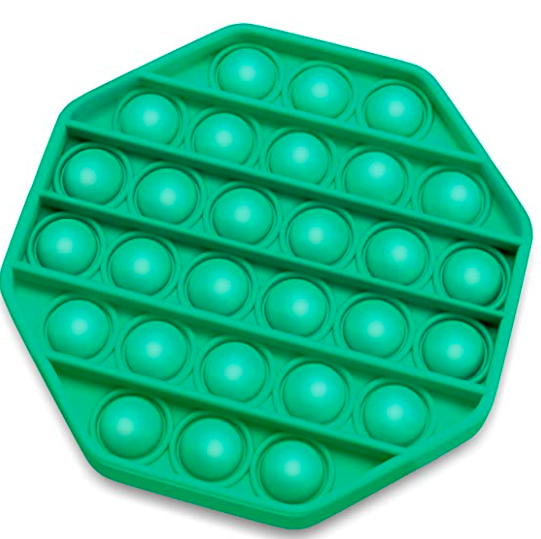
A few important differences to note:

• There are a lot fewer buttons.
• The rows are more salient than the columns because of the horizontal stabilizers.
• There actually are not any columns because the buttons don’t align up and down.
• Each row has a different number of buttons from its neighbor.

All in all, this is a fine toy (and admittedly a lot quieter than a Pattern Machine!) but it does not have a design that leads to the kinds of mathematical play I’ve described above.